 | ||||
 |
 |
| ||
| ||||
|
Our lab and experimentsAsymmetric Landau-Zener Tunneling in a Periodic Potential
Abstract: We explored, theoretically and experimentally, the Landau-Zener tunneling between Bloch bands of a Bose-Einstein condensate in an accelerated optical lattice. The optical lattice depth controls the tunneling barrier, while the optical lattice acceleration controls the time dependence of the Hamiltonian. We showed that the mean-field nonlinearity produces an asymmetry for the tunneling probability. More precisely, the tunneling probability from the lower energy adiabatic state to the upper one is enhanced, while the inverse tunneling probability is suppressed. The asymmetry in the tunneling transition probabilities can be explained qualitatively as follows: The nonlinear term of the Schrödinger equation acts as a perturbation whose strength is proportional to the energy level occupation. If the initial state of the condensate in the lattice corresponds to a filled lower level of the state model, then the lower level is shifted upward in energy while the upper level is left unaffected. This reduces the energy gap between the lower and upper levels and enhances the tunneling. On the contrary, if all atoms fill the upper level, then the energy of the upper level is increased while the lower level remains unaffected. This enhances the energy gap and reduces the tunneling. 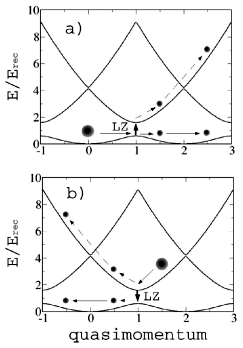 Band structure of a BEC in an optical lattice (V0 = 2 Erec) and LZ tunneling [ground to excited state (a) and excited to ground state (b)]. When the BEC is accelerated across the edge of the Brillouin zone (BZ) at quasimomentum 1, LZ tunneling can occur. Further acceleration will result in the condensate part in the upper level undergoing LZ tunneling to higher bands with a large probability (due to the smaller gaps between higher bands), leaving them essentially unaffected by the lattice. After the first crossing of the edge of the BZ, increasing the lattice depth and decreasing the acceleration leads to a much reduced tunneling rate from the ground-state band at successive BZ-edge crossings.Landau-Zener tunneling between the two lowest energy bands of a condensate inside an optical lattice is investigated in the following way (see Fig. 1). Initially, the condensate is loaded adiabatically into one of the two bands. Subsequently, the lattice is accelerated in such a way that the condensate crosses the edge of the Brillouin zone once, resulting in a finite probability for tunneling into the other band (higherlying bands can be safely neglected as their energy separation at the edge of the Brillouin zone is much larger than the band gap). After the tunneling event, the two bands have populations reflecting the Landau-Zener tunneling rate (assuming that, initially, the condensate populated one band exclusively). In order to experimentally determine the number of atoms in the two bands, we then increase the lattice depth (from 2 Erec to 4 Erec) and decrease the acceleration (from 3 m/s2 to 2 m/s2). In this way, successive crossings of the band edge will result in a much reduced Landau-Zener tunneling probability (of order a few percent). The fraction of the condensate that after the first tunneling event populated the ground-state band will, therefore, remain in that band, whereas the population of the first excited band will undergo tunneling to the second excited band with a large probability (around 90%) as the relevant gap is smaller by a factor of 5 for our parameters. Once the atoms have tunneled into the second excited band, they essentially behave as free particles since higherlying band gaps are smaller still, so they will no longer be accelerated by the lattice. In summary, using this experimental sequence we selectively accelerate that part of the condensate further that populates the ground-state band. In practice, in order to get a good separation between the two condensate parts after a time of flight, we accelerate the lattice to a final velocity of 4 - 6 vrec [14] and absorptively image the condensate after 22 ms. 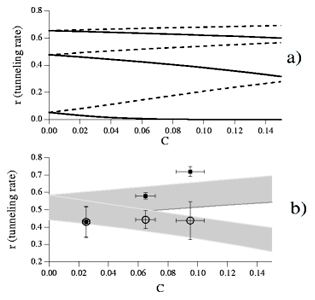 LZ tunneling rate r within the two-level model as a function of the nonlinear parameter C for different accelerations. Results are for V0 = 2.2 Erec, in (a) for aL = 0.8; 3.2; 5.6 m/s2 from bottom to top, and in (b) for aL = 2.9 m/s2. In (a), the dashed (solid) lines correspond to tunneling from the excited (ground-state) band. In (b) experimental results denoted by open (filled) symbols correspond to tunneling from the excited (ground state) band. In summary, we have numerically simulated Landau-Zener tunneling between two energy bands in a periodic potential and found that, in the presence of a nonlinear interaction term, an asymmetry in the tunneling rates arises. Experimentally, we have measured these tunneling rates for different values of the interaction parameter and found qualitative agreement with the simulations. Future experiments could probe the complicated and time-dependent tunneling behavior due to the changing tunneling rate for multiple crossings of the zone edge. Furthermore, when the two lowest bands are initially equally populated, the tunneling behavior should again be linear. We also note here that the phenomenon of asymmetric tunneling should be a rather general feature of quantum systems exhibiting a nonlinearity. For instance, calculating the energy shift due to a nonlinearity for two adjacent levels of a harmonic oscillator, one finds that both levels are shifted upwards in energy, the shift being proportional to the population of the respective level. The energy difference between the levels, therefore, decreases if only the lower state is populated and increases if all the population is in the upper level.
|
|||
|
For comments contact the web master: usala@df.unipi.it |
||||
