Our lab and experiments

Photoionization of a Bose-Einstein condensate

Photoionization of cold atomic samples links together such distinct fields as coherent atom optics, atomic physics, plasma physics and quantum statistics. The excess energy of the ionization fragments can be experimentally controlled and made very small, offering intriguing possibilities to observe collective effects at extremely low temperatures. Close enough to threshold, even the quantum statistics of the products, which are Fermions produced out of quantum degenerate Bosons, may influence the ionization rate itself. Studying the physics of charged particles in coherent clouds experimentally requires controlled production of electron-ion pairs, i.e. accurate knowledge of ionization cross sections and of possible systematic effects different from ionization caused by the applied laser radiation. We have performed experiments on the effects of ionizing laser radiation on thermal clouds and condensates stored inside a magnetic trap are presented.

A. Single ion in a dense and cold cloud

Suppose that an ion is introduced into a dielectric medium. The strong electric field due to the ion charge polarizes the surrounding medium. As a consequence, the surrounding medium experiences a compression with the polarized particles attracted towards regions of higher electric field. For the case of liquid helium, the high compression leads to a helium solidification within a small sphere surrounding the ion. A similar local deformation should take place also for a gaseous Bose-Einstein condensate. The polarization effect is important for the condensate dynamics and may even produce a destruction of the condensate phase. Estimating the global importance of the ion charge in the condensate is less straightforward since the cloud-averaged interaction energy is highly sensitive to the cut-off at small distances due to the singular behavior of the potential between ion and condensate. The observable effects of the presence of a charge inside the condensate depends on the interaction time. In principle the interaction time will be limited by the recoil of the ion and by the condensate size. Experimentally, stray electric or external applied electric fields will limit the effective interaction time to smaller values. In fact, for a laser frequency 100 GHz above threshold and for an ion mass large compared to the electron mass, the ion velocity is 7.6x10-2 m/s, while the electron velocity is about 1.2x104 m/s. For typical condensate dimensions of 5 mm, the corresponding interaction times are 0.4 ns for the electron and 66 ms for the ion. A stray field of 1V/cm will shorten the transversal time for the ion already to 300 ns and to get to interaction times above 10 ms a field below 1 mV/cm is needed.

B. Scattering and dipole force

Apart from changing the internal atomic state the ionizing laser radiation also acts on the center of mass motion of the atoms through the scattering and dipole forces. For ionization above threshold the excess energy of the photon is converted into kinetic energy of the fragments imparting large momentum on the electron and ion. However, for trap loss spectroscopy only the radiation forces acting on the atoms remaining in bound states within the trap need to be considered for additional loss mechanisms. The dissipative environment of a MOT with a typical effective trap depth of 300 mK safely recaptures and cools atoms with velocities of some 10 m/s, while in a conservative magnetic trap with typical depth of 50 mK already atoms accelerated to 10 cm/s can escape from the trap. The relative role of the scattering and dipole forces acting on the atoms depends mainly on the laser detuning from the atomic transitions. For most of our investigations with pulsed lasers, the laser detuning was so large that the scattering forces could be neglected. For the case of a condensate there is a subtle difference between the effects of the scattering and dipole forces. In a spontaneous emission event localizing an atom to within a laser wavelength, the photon recoil is picked up by this atom, as long as the condensate sound velocity is smaller than the atomic recoil velocity. However, the dipole force due to the coherent redistribution of laser photons acts on the whole condensate cloud. For a constant intensity gradient over the condensate, the center of mass motion of the condensate will be driven by the dipole force without internal excitation. By contrast, for laser beam sizes comparable to the condensate size, the gradient of dipole force produces also internal excitations of the condensate. For the impulse approximation in the case of pulsed radiation, a complicated phase pattern is imprinted onto the condensate cloud, which subsequently evolves into considerable modifications of the condensate density distribution, catalyzing the formation of solitons, or splitting the condensate cloud in pieces. Part of these fragments may acquire a high enough kinetic energy to leave the magnetic trap potential, hence leading to additional trap losses.

C. Experimental setup

The energy threshold for ionization of ground state atoms is at 4.17717 eV. Considering a single photon process, the continuum can be reached by one photon of wavelength = 2968.15 Å in vacuum. Alternatively a ground state atom can be ionized through a two-photon process (with no resonant intermediate level), where the wavelength of the ionizing light is = 5936.30 Å in vacuum. The third possibility which has been explored is a two-photon transition excitation to the continuum with the first steep nearly resonant to an atomic level. Choosing the 6P_1/2 or 6P_3/2 hyperfine state, the radiations needed to photoionize a ground state atom have the resonant wavelengths = 4220 Å for the |5S>|6P> transition and = 10020 Å for the final step to the continuum |6P>|c>.

D. Magnetically trapped atoms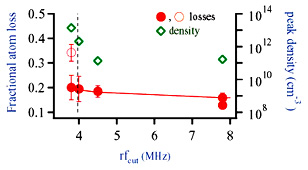

Figure 1:
Fractional loss of atoms after exposure to 120 pulses of the 296 nm laser inside the magnetic trap (filled circles) as a function of the final frequency for RF evaporation (for the open circle data point a longer time of flight was used). The decreasing RF cut produced an increasing atomic peak density (open diamonds). The dashed line indicates the condensation threshold position. The continuous line models the effect of the changing size of the cloud within the spatial distribution of the uv beam.






In the ionization experiment performed with uv light at 296 nm the lower peak intensity (70 mJ) and the large beam size lead to a single pulse ionization probability of 0.002. Because a single pulse produced a loss of atoms too small to be directly detected, we illuminated the magnetically trapped atoms (condensate and non-condensate) with a sequence of 120 pulses, at a repetition rate of 11 Hz. We performed the investigation of the ionization losses starting with an initial cold cloud of 10⁶ atoms and, varying the final rf frequency cut, we ionized clouds with decreasing temperature and increasing atom density. Finally, for radio-frequency cuts below the 3.95 MHz threshold, we ionized a pure condensate. Data for the peak density and the fractional atom loss are reported in fig.1 as a function of the final rf value. The measured total fractional losses between 15 and 20% were compared to the single pulse loss extracted from the MOT measurements described by the continuous curve in fig. 1 taking into account the changing size of the atomic cloud and an overall scale factor of 1.5, due to an estimated misalignement of 100 mm between the atomic cloud and the laser beam. We verified that the temperatures of the thermal clouds were unaffected by the laser pulses within the experimental uncertainty of 5%. However, when using a longer time of flight before imaging for a pure Bose condensed cloud (in order to decrease the optical density, thus getting more reliable data for the number of atoms), we measured higher losses (the open circle in fig.1). This result indicates the presence of very low energy thermal atoms removed from the condensate but still trapped, albeit with a spatial density below our detection sensitivity. Thus the 296 nm laser acting on a condensate produces additional loss of low energy thermal atoms ejected from the condensate cloud. Since for the large beam size and the moderate pulse energy we did not expect a significant contribution of scattering and dipole forces, we have taken these additional losses as first evidence for the interaction of ionization products with the condensate background.
Figure 2:
2-photon ionization loss rate for a single laser pulse, normalized by the pulse energy, as a function of the dye-laser wavelength. The vertical line indicates the threshold wavelength for the 2-photon ionization from the ground state.











Using the 594 nm pulsed radiation, we irradiated the magnetically trapped atoms either with few pulses or by applying a single laser pulse. The puzzling result of these investigations was the measurement of a large loss rate, larger by a factor ten than the value expected from the previously measured 2-photon ionization cross-section. Moreover, the measured trap losses depended strongly on the alignment of the photoionizing laser with respect to the magnetic trap. For perfect alignment and small clouds we expected an ionized fraction of 2%, while losses of 20% were observed. An example of the measured fractional loss of atoms from the magnetic trap, normalized to the laser pulse energy, is reported in fig.2 as a function of the wavelength of the ionizing laser. After the laser pulse, the magnetic trap remains on for t_trap=20.2 ms and the cloud is imaged after a time of flight of 18 ms. Looking at the cloud size, we do not see appreciable enlargement due to heating after the laser pulses application. The data of fig.2 do not exhibit a clear evidence of threshold behavior, similarly to the behavior of the atoms in the MOT. In addition, in a magnetic trap other loss mechanisms are possible: in fact, every process changing the hyperfine or m-sublevel leads to atom loss. These Raman scattering processes do not produce losses in a MOT, since in a magneto-optical trap atoms belonging to every Zeeman sublevel of the fundamental state are trapped and the kinetic energy acquired in a Raman scattering is smaller than the trap depth.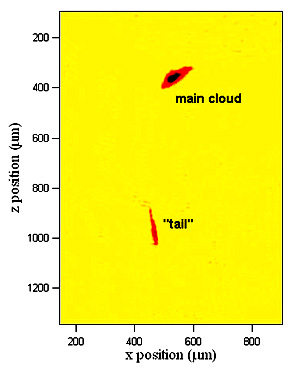

Figure 3:
Shadow image of a condensate after the application a single dye laser pulse at 594 nm. The condensate is deformed and a secondary condensate containing around 30% of the original atoms is present (the periodic circular fringe patterns are an artifact of the imaging technique). The size of the main cloud at the ionization time was 7 mm, the trapping frequency 23 Hz, the time of flight 18 ms.























The presence of more complex processes is indicated also by the spatial distribution of the atoms after the application of the laser pulse at 594 nm. For both cases of thermal and condensed clouds, the remaining atoms exhibit a very irregular shape. We noticed that the photoionization pulse ejected a secondary cloud, a "tail", from the original one (see fig. 3). The shadow image of fig. 3 is obtained after 11 ms evolution of the condensates within the magnetic trap and a 18 ms free fall time of flight, and shows two condensate clouds, the upper cloud at the place of the original one and the ejected secondary cloud separated in space, at a lower vertical position. We observed that the secondary cloud contains around the 30% of the total number of atoms, but in other conditions this fraction can reach the 50%. Its position, after a fixed time of flight, depends on the duration of the free evolution after the pulse inside the trap.
Measuring the atomic density and temperature for the two clouds separately we derived that the phase space density of the ejected cloud was comparable to the remaining fraction of the original cloud. Below the condensation threshold both remaining clouds had a phase space density far above 1. This suggests that the laser pulse splits the original condensate into two condensed clouds. Both the observed clouds were strongly deformed with respect to the unperturbed condensate, which shows a close to spherical density distribution after the same time of flight. The aspect ratio and orientation of the deformed cloud depended on the evolution time within the magnetic trap after the laser excitation. Moreover a detailed analysis, outlined below, showed for the main cloud a negligible center of mass motion, while the secondary cloud acquired a center of mass motion during the interaction with the laser pulse.

E. Interpretation of the "tail"

In order to understand the nature of the secondary cloud, we measured the position of the secondary condensate as a function of the evolution time t _magn within the magnetic trap after the application of the laser pulse. From the oscillatory behavior of the difference in position of the two clouds we inferred that the secondary cloud consisted of rubidium atoms in the | F=2,m_F=1> Zeeman state, excluding the | F=1,m_F=-1> state with similar magnetic moment, because our detection was sensitive only to atoms in the F=2 state. The absence of atoms in the |F=1> hyperfine states has been tested by applying a repumper laser before imaging the primary and secondary clouds and verifying that the atom numbers in the clouds were not modified. The equilibrium position of the | F=2,m_F=1> condensate within the magnetic trap is different from that of the | F=2,m_F=2> condensate, because of the different magnetic moment of this state (m_F=2=m_B, while m_F=1=m_B /2). The | F=2,m_F=1> condensate is created in the magnetic trap equilibrium position of the | F=2,m_F=2> condensate, so it starts to oscillate. A fit to the measured position data, assuming that the secondary cloud was created at the instant of the laser pulse from the position of the mother condensate, revealed that the secondary cloud started its oscillation with an initial velocity of


i.e. a velocity pointing downwards along the vertical axis.

F. Conclusions and outlook

We applied trap loss spectroscopy to measure the photoionization cross-sections of rubidium atoms confined in a MOT making use of cw and pulsed laser. We verified that the use of pulsed lasers did not limit the accuracy reached in the cross-section determinations. We demonstrated that the photoionization of magnetically trapped thermal and condensed atoms instead does not represent a precise tool for cross-section determinations. In effect the magnetic trap depth is smaller than the MOT depth, and the laser action on the cold atomic cloud may originate additional processes leading to atomic losses from the trap, masking the ionization losses. We have observed that both scattering and dipole forces associated with strong pulsed lasers may impart a kinetic energy large enough to overcome the trap depth. Moreover dealing with a condensate cloud, additional losses appear that are probably due to the creation of a dilute thermal cloud. We have discovered that the application of intense pulsed radiation led to huge excitation and macroscopic splitting of a condensate without compromising its phase space density. We identified the transfer of atoms to the | F=2, m_F=1 > level as an important process, but the detailed microscopic mechanism remains unclear and will be subject of further studies.
On the basis of the photoionization cross-sections measured within a MOT we estimated to have created charged clouds and condensates in magnetic traps containing a fraction of 0.2% and 2% of ions at a time for the 1-photon and 2-photon experiments respectively. We have not observed any dramatic instability which could be triggered by the strong polarization of those charged condensates. However, we take the higher losses measured following the 1-photon ionization of a condensate as a first indication of the interaction between the ionization products and the condensate background. Additional processes such as collisions with cold electrons and ultra-cold neutrals, recombination and collective processes in the weak and cold plasma could modify the atomic cloud evolution and in part be responsible for the more complicated dynamics observed after the 2-photon excitation in the condensate. In future experiments we plan to use cw sources to ionize condensed clouds to disentangle scattering and dipole force effects from the rich and interesting physics of cold charged particles in quantum degenerate clouds.

For further reading, please see

• D. Ciampini, M. Anderlini, J. H. M¸ller, F. Fuso, O. Morsch, J. W. Thomsen and E. Arimondo, "Photoionization of ultracold and Bose-Einstein-condensed Rb atoms", Phys. Rev. A 66, 043409 (2002).
• R. Cote', V. Kharchenko and M. D. Lukin , "Mesoscopic Molecular Ions in Bose-Einstein Condensates", Phys. Rev. Lett. 89, 093001 (2002).
• Also see: http://focus.aps.org/story/v10/st8

---

File translated from T_EX by T_TH, version 3.30.
On 17 Jan 2003, 12:25.